Solving Equations Containing
Rational Expressions
Example 1
Solve:
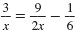
Solution
| Step 1 Find the LCD.
|
LCD = 2 · 3 · x = 6x |
| Step 2 Multiply by the LCD. |
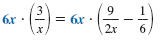 |
| Step 3 Distribute on the right side.
|
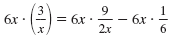 |
| Step 4 Reduce. |
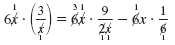 |
| Step 5 Solve.
|
|
| Simplify.
Subtract 27 from both sides.
Multiply both sides by -1. |
18 = 27 - x -9 = -x
9 = x |
| Step 6 Check the solution.
We leave the check to you.
|
|
| Thus, the solution is x = 9. |
|
Note:
Here’s one way to find the LCD of
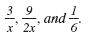
• Factor each
denominator:
x = x
2x = 2 · x
6 = 2 · 3
• List each factor the
greatest number of
times it appears in
any factorization. x, 2, 3
• Multiply. 2 · 3
· x = 6x
So, the LCD is 6x.
Example 2
Solve:
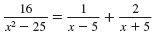
Solution
| Step 1 Find the LCD.
|
LCD = (x - 5)(x + 5) |
| Step 2 Multiply by the LCD. |
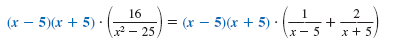 |
| Step 3 Distribute the LCD to both terms
the right side. |
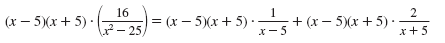 |
| Step 4 Reduce. |
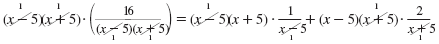 |
| Step 5 Solve.
|
|
| Simplify.
Distribute on the right side.
Combine like terms.
Add 5 to both sides.
Divide both sides by 3.
|
16
16
16
21
7 |
= x + 5 + (x - 5) · 2
= x + 5 + 2x - 10
= 3x - 5
= 3x
= x |
| Step 6 Check the solution.
We leave the check to you.
|
|
Thus, the solution is x = 7.
|