Solving Quadratic Equations by Factoring
Example
Solve by factoring: 25 + 30x = -9x2
Solution
| Step 1 Write the equation in the form
ax2 + bx + c = 0 Add 9x2 to both sides.
Step 2 Factor the polynomial.
The first and last terms of the
trinomial are perfect squares.
The middle term, 30x, is 2(3x)(5).
Since the trinomial has the form
a2 + 2ab + b2, it is a perfect
|
9x2 + 30x +
25 (3x)2 + 30x + (5)2 (3x)2
+ 2(3x)(5) + (5)2 |
= 0 = 0 = 0 |
| A perfect square trinomial can be
written as the product of two
identical binomials. |
(3x + 5)(3x + 5) |
= 0 |
| Step 3 Use the Zero Product Property. |
3x + 5 = 0 or 3x + 5 |
= 0 |
| Step 4 Solve the resulting equations.
Step 5 Check each answer.
We leave the check to you. |
 |
So, 25 + 30x = -9x2 has two equal solutions,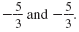
Every quadratic equation has two solutions.
In the previous example, the two solutions are the same,
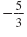 . We call . We call
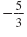 a solution of
multiplicity two. a solution of
multiplicity two.
|